NOTE: The data shown here is based on the 2021 glacier update at Mount Rainier, and should be considered the most up-to-date data on glaciers at the park (2023-04-06).
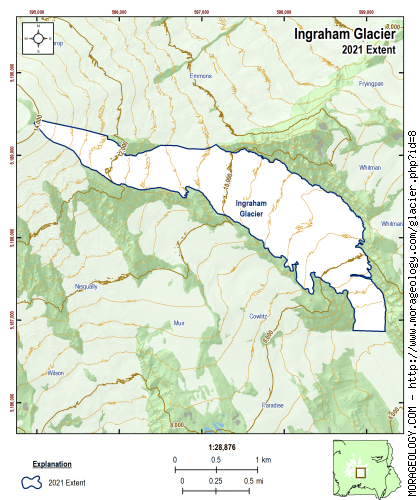
Ingraham Glacier
Ingraham Glacier, on the southeast slope of Mount Rainier, was named by Professor I.C. Russell in 1896 for Edward Sturgis Ingraham of Seattle, an early mountaineer. Ingraham arrived in Seattle in 1875 from Maine. He taught school and was Superintendent of King County Schools from 1875 to 1882 and of Seattle Schools from 1883 to 1889. He was a Colonel in the Militia, but was usually referred to as Major Ingraham. He climbed Mount Rainier eleven times often serving as a guide. He died in Seattle August 16, 1926. (Reese, 2009)
Switch Glacier:
|
STATISTICS AS OF 2021 (Beason et al., 2023):
Aerial Extent:
1.144 ± 0.063 square miles
(2.962 ± 0.162 km2)
(Rank: 11 of 29)
Debris Cover - Based on data from 2015 (Beason, 2017):
0.106 ± 0.003 square miles
(0.276 ± 0.007 km2)
(Rank: 12 of 29)
Estimated Glacier Volume: (See notes below)
0.0294 ± 0.0103 cubic miles
(0.1224 ± 0.0428 km3)
Highest Elevation (Head):
14,064 feet
(4,287 m)
Lowest Elevation (Terminus):
6,493 feet
(1,979 m)
Elevation Range:
7,572 feet
(2,308 m)
Length:
3.22 miles
(5.18 km)
Average Slope:
25.17°
Average Flow Direction (direction the glacier flows towards):
East southeast (120°)
Glacier Type:
S - Glacier Head Near Summit |
|
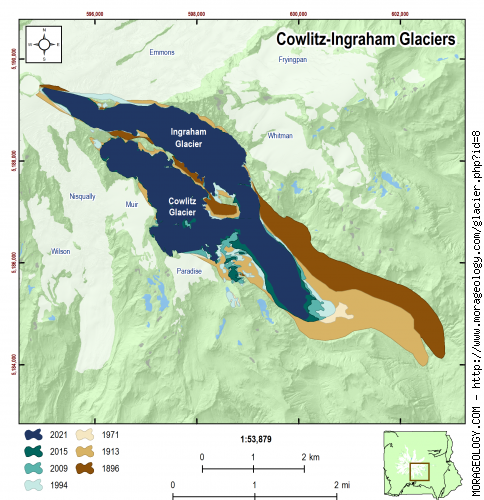
CHANGE IN EXTENT, 1896-2021 (Beason et al., 2023):
| Units |
1896 |
1913 |
1971 |
1994 |
2009 |
2015 |
2021 |
| Area, mi2 |
-- |
-- |
-- |
-- |
-- |
1.161 |
1.144 |
| Area, km2 |
-- |
-- |
-- |
-- |
-- |
3.007 |
2.962 |
Area Change Between Periods
|
1913 |
1971 |
1994 |
2009 |
2015 |
2021 |
| 1896 | -- | -- | -- | -- | -- | -- |
|---|
| 1913 | -- | -- | -- | -- | -- | -- |
|---|
| 1971 | | -- | -- | -- | -- | -- |
|---|
| 1994 | | | -- | -- | -- | -- |
|---|
| 2009 | | | | -- | -- | -- |
|---|
| 2015 | | | | | -- | -0.017 mi2
(-0.045 km2) |
|---|
Percent Change Between Periods
|
1913 |
1971 |
1994 |
2009 |
2015 |
2021 |
| 1896 | -- | -- | -- | -- | -- | -- |
|---|
| 1913 | -- | -- | -- | -- | -- | -- |
|---|
| 1971 | | -- | -- | -- | -- | -- |
|---|
| 1994 | | | -- | -- | -- | -- |
|---|
| 2009 | | | | -- | -- | -- |
|---|
| 2015 | | | | | -- | -1.49% |
|---|
|
|
ESTIMATED CHANGE IN VOLUME, 1896-2021 (Beason et al., 2023):
PLEASE see important notes about this, below...
Glacier-specific Scaling Parameter, c:
0.029899
| Units |
1896 |
1913 |
1971 |
1994 |
2009 |
2015 |
2021 |
| Volume, mi3 |
-- |
-- |
-- |
-- |
-- |
0.0300 |
0.0294 |
| Volume, km3 |
-- |
-- |
-- |
-- |
-- |
0.1249 |
0.1224 |
Volume Change Between Periods
|
1913 |
1971 |
1994 |
2009 |
2015 |
2021 |
| 1896 | -- | -- | -- | -- | -- | -- |
|---|
| 1913 | -- | -- | -- | -- | -- | -- |
|---|
| 1971 | | -- | -- | -- | -- | -- |
|---|
| 1994 | | | -- | -- | -- | -- |
|---|
| 2009 | | | | -- | -- | -- |
|---|
| 2015 | | | | | -- | -0.001 mi3
(-0.003 km3) |
|---|
Percent Change Between Periods
|
1913 |
1971 |
1994 |
2009 |
2015 |
2021 |
| 1896 | -- | -- | -- | -- | -- | -- |
|---|
| 1913 | -- | -- | -- | -- | -- | -- |
|---|
| 1971 | | -- | -- | -- | -- | -- |
|---|
| 1994 | | | -- | -- | -- | -- |
|---|
| 2009 | | | | -- | -- | -- |
|---|
| 2015 | | | | | -- | -2.04% |
|---|
Important comments about the calculation of volume shown here
The calculation of glacial volume shown on this page is based on an analysis of two methods used at Mount Rainier in the past (Driedger and Kennard [1986]; and Nylen [2001]) as well as the most recent literature review for glacier area-volume scaling (Please review Beason et al. [2023] for an in-depth discussion about this issue). It should be noted that simply converting area to volume with an equation is extremely difficult and the values presented here have extremely large error margins (likely ± 35% or more). With that in mind, the values presented here should give you an estimate of the glacial volume and change in volume over time. Please use these data very carefully with those caveats.
The calcuation of the volume is as follows:
\[V_i = {(c_iA_i^{1.375}) + (c_nA_i^{1.36}) \over 2}\]
Where:
\(V_i\) = Average volume for the glacier in question (km3);
\(c_i\) = The glacier-specific scaling parameter (back-calculated from glacier area and volume in 1971 in Driedger and Kennard (1986); Method described in Beason et al. (2023). The value for the Ingraham Glacier is 0.029899 (this is also listed above the volume graph);
\(c_n\) = The back-calculated scaling parameter from Nylen (2001) of 0.0255; and
\(A_i\) = The measured volume of the glacier in question (km2).
This is essentially an average of the back-calculated Dreidger and Kennard (1986) and Nylen (2001) methods (D&K is in the first parenthesis; Nylen in the second). For example, for the Ingraham Glacier in 2021, you can find the following individual volumes:
Back-calculated Dreidger and Kennard (1986) Method: 0.0319 mi3 (0.1331 km3).
Back-calculated Nylen (2001) Method: 0.0268 mi3 (0.1117 km3).
Average of the two (above equation and values listed for 2021 here): 0.0294 mi3 (0.1224 km3).
Official volume estimate listed above, with error: 0.0294 ± 0.0103 mi3 (0.1224 ± 0.0428 km3).
As you can see, the D&K method tends to produce higher values and Nylen produces lower values; the average of these two methods probably estimates the glacial volume. Until further research is done in this area and we can develop a better method or equation to determine volumes, this is the method we are using to determine glacial volumes. For more information about this method, please read the methods section of Beason et al. (2023).
|
NOTES:
This glacier flows into the Cowlitz Glacier. Extent of this glacier from 1896-2009 was included with the combined Cowlitz/Ingraham Glacier system. See Cowlitz Glacier for extent change over time.
RAW DATA: